Cho tứ giác ABCD. Tổng số đo các góc ngoài tại 4 đỉnh A, B, — Không quảng cáo

Phương pháp giải

Sử dụng góc ngoài và góc trong tứ giác tại một đỉnh là hai góc kề bù.

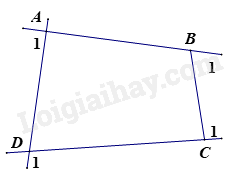
Gọi góc ngoài tại 4 đỉnh A, B, C, D của tứ giác ABCD lần lượt là: $\widehat {{A_1}};\widehat {{B_1}};\widehat {{C_1}};\widehat {{D_1}}$ .

Khi đó ta có:

$\begin{array}{l}\widehat A + \widehat {{A_1}} = {180^o} \Rightarrow \widehat {{A_1}} = {180^o} - \widehat A\\\widehat B + \widehat {{B_1}} = {180^o} \Rightarrow \widehat {{B_1}} = {180^o} - \widehat B\\\widehat C + \widehat {{C_1}} = {180^o} \Rightarrow \widehat {{C_1}} = {180^o} - \widehat C\\\widehat D + \widehat {{D_1}} = {180^o} \Rightarrow \widehat {{D_1}} = {180^o} - \widehat D\end{array}$

Suy ra:

$\begin{array}{l}\widehat {{A_1}} + \widehat {{B_1}} + \widehat {{C_1}} + \widehat {{D_1}} = \left( {{{180}^o} - \widehat A} \right) + \left( {{{180}^o} - \widehat B} \right) + \left( {{{180}^o} - \widehat C} \right) + \left( {{{180}^o} - \widehat D} \right)\\\widehat {{A_1}} + \widehat {{B_1}} + \widehat {{C_1}} + \widehat {{D_1}} = {720^o} - \left( {\widehat A + \widehat B + \widehat C + \widehat D} \right)\\\widehat {{A_1}} + \widehat {{B_1}} + \widehat {{C_1}} + \widehat {{D_1}} = {720^o} - {360^o} = {360^o}\end{array}$

Vậy số đo 4 góc ngoài tứ giác tại 4 đỉnh A, B, C, D bằng ${360^o}$