Bài 1, 2, 3, 4 trang 137 SGK Toán 4 — Không quảng cáo

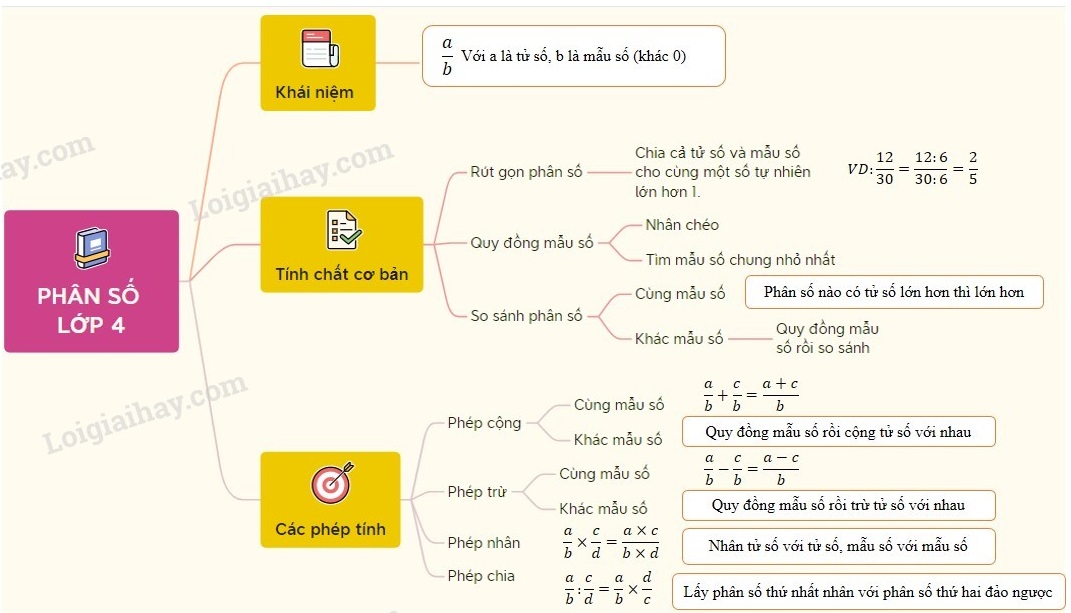
Sơ đồ tư duy: Phân số lớp 4

Bài 1

Tính rồi rút gọn:

a) $\displaystyle {2 \over 7}:{4 \over 5};$                                 b) $\displaystyle {3 \over 8}:{9 \over 4};$

c) $\displaystyle {8 \over {21}}:{4 \over 7};$                               d) $\displaystyle {5 \over 8}:{{15} \over 8}$

Phương pháp giải:

Muốn chia hai phân số ta lấy phân số thứ nhất nhân với phân số thứ hai đảo ngược.

Lời giải chi tiết:

a) $\displaystyle{2 \over 7}:{4 \over 5} = {2 \over 7} \times {5 \over 4} = {{10} \over {28}} = {{10:2} \over {28:2}}$$\displaystyle= {5 \over {14}};$

Hoặc : $\displaystyle{2 \over 7}:{4 \over 5} = {2 \over 7} \times {5 \over 4} = {{2 \times 5 } \over {7 \times 4 }}$$\displaystyle= {{2\times  5} \over {7 \times  2 \times  2}} = {5 \over {14}};$

b) $\displaystyle{3 \over 8}:{9 \over 4} = {3 \over 8} \times {4 \over 9} = {{12} \over {72}} = {{12:12} \over {72:12}}$$\displaystyle = {1 \over 6};$

Hoặc : $\displaystyle{3 \over 8}:{9 \over 4} = {3 \over 8} \times {4 \over 9} = {{3\times 4 } \over {8 \times  9}}$$\displaystyle = {{3 \times  4} \over {4 \times  2 \times  3 \times  3}} = {1 \over 6};$

c) $\displaystyle{8 \over {21}}:{4 \over 7} = {8 \over {21}} \times {7 \over 4} = {{56} \over {84}}$ $\displaystyle= {{56:28} \over {84:28}}  = {2 \over 3};$

Hoặc : $\displaystyle{8 \over {21}}:{4 \over 7} = {8 \over {21}} \times {7 \over 4} = {{8\times  7} \over {21 \times  4}}$ $\displaystyle= {{2 \times  4 \times  7} \over {3 \times  7 \times  4}}  = {2 \over 3};$

d) $\displaystyle{5 \over 8}:{{15} \over 8} = {5 \over 8} \times {8 \over {15}} = {{40} \over {120}}$ $\displaystyle = {{40:40} \over {120:40}}  = {1 \over 3}.$

Hoặc :  $\displaystyle{5 \over 8}:{{15} \over 8} = {5 \over 8} \times {8 \over {15}} = {{5 \times  8} \over {8 \times  15}}$ $\displaystyle = {{5 \times 8} \over {8 \times  5 \times  3}}  = {1 \over 3}.$

Bài 2

Tính (theo mẫu):

Mẫu: $\displaystyle 2:{3 \over 4} = {2 \over 1} : { 3 \over 4} = { 2\over 1} \times {4 \over 3 }= {8 \over 3}$

Ta có thể viết gọn như sau: $\displaystyle 2:{3 \over 4} = {{2 \times 4} \over 3} = {{8} \over 3}$

a) $\displaystyle3:{5 \over 7};$                              b) $\displaystyle4:{1 \over 3};$                             c) $\displaystyle5:{1 \over 6}.$

Phương pháp giải:

Để chia số tự nhiên cho phân số ta có thể viết số tự nhiên dưới dạng phân số có mẫu số là $1$, sau đó thực hiện phép chia hai phân số như thông thường; hoặc ta viết gọn lại tương tự như ở ví dụ mẫu.

Lời giải chi tiết:

$\displaystyle\eqalign{ & a)\,\,3:{5 \over 7} = {{3 \times 7} \over 5} = {{21} \over 5}; \cr & b)\,\,4:{1 \over 3} = {{4 \times 3} \over 1} = 12; \cr & c)\,\,5:{1 \over 6} = {{5 \times 6} \over 1} = 30. \cr}$

Bài 3

Tính bằng hai cách:

$\displaystyle a)\,\,\left( {{1 \over 3} + {1 \over 5}} \right) \times {1 \over 2};$                                $\displaystyle  b)\,\,\left( {{1 \over 3} - {1 \over 5}} \right) \times {1 \over 2}.$

Phương pháp giải:

Cách 1: biểu thức có dấu ngoặc thì ta tính trong ngoặc trước, ngoài ngoặc sau.

Cách 2: Áp dụng công thức nhân một tổng hoặc một hiệu với một số:

$(a+b)\times c = a \times c + b \times c$  ;                   $(a-b)\times c = a \times c - b \times c$

Lời giải chi tiết:

a) Cách 1:

$\displaystyle\,\,\left( {{1 \over 3} + {1 \over 5}} \right) \times {1 \over 2} = \left( {{5 \over {15}} + {3 \over {15}}} \right) \times {1 \over 2}$

$\displaystyle= {8 \over {15}} \times {1 \over 2}  = {8 \over {30}}= {4 \over {15}};$

Cách 2:

$\displaystyle\,\,\left( {{1 \over 3} + {1 \over 5}} \right) \times {1 \over 2} = {1 \over 3} \times {1 \over 2} + {1 \over 5} \times {1 \over 2}$

$\displaystyle= {1 \over 6} + {1 \over {10}} = {{10} \over {60}} + {6 \over {60}} = {{16} \over {60}} = {4 \over {15}}$

b) Cách 1:

$\displaystyle\,\left( {{1 \over 3} - {1 \over 5}} \right) \times {1 \over 2} = \left( {{5 \over {15}} - {3 \over {15}}} \right) \times {1 \over 2}$

$\displaystyle= {2 \over {15}} \times {1 \over 2}  = {2 \over {30}}= {1 \over {15}}$

Cách 2:

$\displaystyle\left( {{1 \over 3} - {1 \over 5}} \right) \times {1 \over 2} = {1 \over 3} \times {1 \over 2} - {1 \over 5} \times {1 \over 2}$

$\displaystyle= {1 \over 6} - {1 \over {10}}$$\displaystyle= {{10} \over {60}} - {6 \over {60}}$$\displaystyle=  {4 \over {60}}  = {1 \over {15}}$

Bài 4

Cho các phân số $\displaystyle{1 \over 2}\,;\;{1 \over 3}\,;\;{1 \over 4}\,;\;{1 \over 6}$. Hỏi mỗi phân số đó gấp mấy lần $\displaystyle{1 \over {12}}$?

Mẫu: $\displaystyle{1 \over 2}:{1 \over {12}} = {1 \over 2} \times {{12} \over 1} = {{12} \over 2} = 6$

Vậy: $\displaystyle{1 \over 2}$ gấp 6 lần $\displaystyle{1 \over {12}}$.

Phương pháp giải:

Thực hiện phép chia hai phân số để tìm thương của hai phân số đó.

Lời giải chi tiết:

+) $\displaystyle{1 \over 3}:{1 \over {12}} = {1 \over 3} \times {{12} \over 1} = {{12} \over 3} = 4$

Vậy: $\displaystyle{1 \over 3}$ gấp $4$ lần $\displaystyle{1 \over {12}}$.

+) $\displaystyle{1 \over 4}:{1 \over {12}} = {1 \over 4} \times {{12} \over 1} = {{12} \over 4} = 3$

Vậy: $\displaystyle{1 \over 4}$ gấp $3$ lần $\displaystyle{1 \over {12}}$.

+) $\displaystyle{1 \over 6}:{1 \over {12}} = {1 \over 6} \times {{12} \over 1} = {{12} \over 6} = 2$

Vậy: $\displaystyle{1 \over 6}$ gấp $2$ lần $\displaystyle{1 \over {12}}$.